Signals & Systems Projects

Project 1：Matched filtering of Linear Frequency Modulated (LFM) signal 线性调频信号的匹配滤波

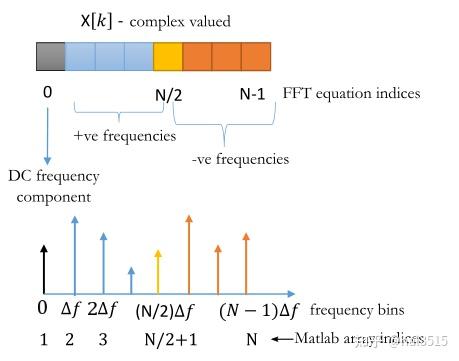
FFT function

fig01

Parameters: 1. sampling time: 2. sampling rate: 3. 4. frequency index: 5. original index: (same as 3.)

Generating Original Waves

According to the Euler's formula, we can get:

The real part of the signal is:

The imaginary part of the signal is:

T=10e-6;                                       
B=40e6;                                        
K=B/T;                                         
Fs=2*B;Ts=1/Fs;                                
N=T/Ts;
t=linspace(-T/2,T/2,N);
St=exp(1i*pi*K*t.^2);
figure(1)
subplot(311)
plot(t*1e6,real(St));
xlabel('time/us');
title('waveform of LFM signal real part');
grid on;axis tight;
subplot(312)
plot(t*1e6,imag(St));
xlabel('time/us');
title('waveform of LFM signal imaginiary part');
grid on;axis tight;
subplot(313)
freq=linspace(-Fs/2,Fs/2,N);
plot(freq*1e-6,fftshift(abs(fft(St))));
xlabel('f/MHz');
title('corresponding amplitude spectrum');
grid on;axis tight;

Matched filtering

St_wgn = awgn(St,10);%%叠加高斯白噪声，SNR= 10
Ht = conj(fliplr(St));%%发射信号时间反褶后取共轭得到h(t)

yt = conv(St_wgn, Ht) ;%%时域信号
yf = Sf.*Hf ;%%频域信号
t_0=linspace(-T/2,T/2,2*N-1);
freq=linspace(-Fs/2,Fs/2,2*N-1);
figure(2)
subplot(311)
plot(t_0*1e6,real(yt));
xlabel('time/us');
title('real part of output signal');
grid on;axis tight;
subplot(312)
plot(t_0*1e6,imag(yt));
xlabel('time/us');
title('imaginary part of output signal');
grid on;axis tight;
subplot(313)
plot(freq*1e-6,fftshift(abs(fft(yt))));
xlabel('f/MHz');
title('corresponding amplitude spectrum of output signal');
grid on;axis tight;

Principle Analysis

考虑观测信号：

为已知信号，为零均值的加性平稳噪声（白色或有色）

• 白噪声（white noise）是指功率谱密度在整个频域内是常数的噪声。 所有频率具有相同能量密度的随机噪声称为白噪声。

• 有色噪声（ coloured noise）是指功率谱密度函数不平坦的噪声。大多数的噪声的频谱主要都是非白色频谱，通过信道的白噪声受信道频率的影响而变为有色的。

令为滤波器的时不变冲激响应函数，目标就是设计滤波器，使得滤波器的输出信号的信噪比最大化。 滤波器的输出信号为：

、分别为滤波器的输出信号分量和噪声信号分量。

在时刻，滤波器的输出信噪比定义为

利用傅里叶变换的卷积特性，有

式中， = 为滤波器的频率响应函数（传递函数）， = 为信号的频谱密度函数。

时刻，输出信号的瞬时功率为

时刻，输出噪声的平均功率为

---

补一些功率谱密度的知识： 若某一个功率信号的功率为，则有

其功率谱密度函数为

若以f为自变量，则可以写成

(其中为的傅里叶变换, 为在上的截断信号) 根据Parseval定理

总功率为：

---

令为加性噪声的功率谱密度函数，则输出噪声的功率谱密度函数为

输出噪声的平均功率可以写作(以频率作为量度)

代入信噪比定义式，可以得出

分子凑了一个, 因为要用到Cauchy-Schwartz不等式：

等号在当且仅当, c是任意复常数时成立。取c = 1：

取最大值，即为等式成立条件。将式中等号成立时的滤波器传递函数记作, 利用Cauchy-Schwartz不等式等号成立条件：

可以得到最大信噪比：

由此，最优线性滤波器理论推导完成。

由题意得其功率谱也恒定为 .代入 中得到：

对其进行反变换，得到最优线性滤波器的时域表达式：

时，最优线性滤波器为：

对应题干中：, 能得到N = 2. 该噪声的功率谱密度为1，该噪声为一个白噪声。

Conclusion

1. 匹配滤波器的单位冲激响应是原信号的共轭反转信号
2. 匹配滤波后的实部输出非常像Sinc Function 原因：输出信号的幅度：

当时，包络近似为辛格（sinc）函数。

3. 输出幅度/功率在处有最大值（考虑延迟，则在）

Radar